The 25% Rule Revisited And A New Investment-Based Method For Determining FRAND Licensing Royalties
Chalmers Univ. of Technology,
Department of Technology Management & Economics,
Professor, Industrial Management and Economics,
Gothenburg, Sweden
Chalmers Univ. of Technology,
Department of Technology Management & Economics,
Researcher, PhD Candidate,
Gothenburg, Sweden
Abstract
This paper starts with briefly discussing the 25% rule and the argumentation for and against it. The paper continues with developing a new investment-based method for determining FRAND licensing royalties, a method not only applicable to one-to-one bilateral licensing deals but also to multilateral deals with multiple license sellers and multiple license buyers. The paper ends with discussing limitations and generalizations, opening up for further research.
1. Introduction
Contemporary technology-based businesses become increasingly dependent on technologies distributed over different technological areas and among different companies and other actors (Granstrand et al., 1997; Granstrand, 1999; Granstrand and Holgersson, forthcoming; Holgersson, 2011). Thus, companies must engage in an increasing amount of technology trade and technology collaborations, leading to various forms of licensing deals (Granstrand, 2004). In this process, methods for royalty determination and value sharing are of utmost importance, and standard bodies then often require their participants to offer Fair, Reasonable, And Non-Discriminatory (FRAND) licensing terms to potential licensees (license buyers). Each of these terms F, R, and ND is ambiguous, however, allowing for a variety of rules and methods to be used, more or less grounded in theory or data.
One method of establishing royalty rates is the 25% rule of thumb, essentially saying that the licensee should pay 25% of operating profits (or sometimes gross profit or EBITDA) to the licensor (license seller) as a standard rate, although a more general version of the rule, the so called classic 25% rule, maintains that this should also be subject to further negotiations and adjustments to case specific factors, as described by Goldscheider (2011), e.g. by utilizing the 15 so called Georgia-Pacific factors. The 25% rule has in recent years attracted a great deal of attention and discussion, not the least in les Nouvelles, about whether it is alive or dead (if it ever lived) and what its destiny should be, especially after the dismissal of the 25% rule of thumb by the United States Court of Appeals for the Federal Circuit (CAFC) in early 2011.1Articles about the rule are then typically polarized into for and against the rule, where the proponents of the rule tend to view it more as a flexible methodology (the classic 25% rule), while the opponents tend to view it more narrow as a rule of thumb (the 25% rule of thumb), see Goldscheider (2012).
While Goldscheider (2011), Kemmerer and Lu (2009), and Lu (2011) argue that empirical evidence suggests that the 25% rule is (still) valid, Kidder and O'Brien (2011) counter-argue that the empirical evidence does not support the rule and that there are theoretical problems with it, e.g. since it does not consider that a product is often not based on only one licensed patent. Further, Epstein and Marcus (2003, p. 574) argue that “the 25% rule assigns royalties without regard to any of the determinants of a reasonable royalty rate.” Although adjustments can (and should) be made to the rule to account for case specific factors, as discussed by Goldscheider (2011), Kidder and O'Brien (2011) discuss the impact an initial “starting point” rate has on the final outcome after adjustments, and argue that utilizing 25% as a standard rate subject to further negotiations and adjustments would impact the final result due to anchoring (see Tversky and Kahneman, 1974) and lead to rates closer to 25% than would otherwise have been the case. Smith and Parr (1998) and Razgaitis (1999) further argue that the 25% rule does not consider relative levels of investments.
So, how should a fact-based reasonable royalty rate be calculated? Epstein and Marcus (2003) argue that the maximum reasonable royalty is the amount that gives a net present value equal to zero for the licensee, considering alternative investments. A similar approach is suggested by Ruikka (2008). This can then act as a starting point for setting a reasonable royalty rate, as it sets a cap for the royalty payment. However, as argued by Sherry and Teece (2004), patents are rarely known to be valid at the time of a licensing negotiation (exceptions being patents that have already been tested in court), and hence the royalty needs to be adjusted for the fact that the patent might not be valid if tested in court, as well as for other factors.
Little emphasis in this literature has been put on the fact that the patents necessary to produce and market a product are many times held by more than one patent holder or licensor, and in addition often licensed to more than one licensee, which makes it meaningless to treat individual licensing deals separately, as argued by e.g. Ruikka (2008). This is especially true in systems technologies, e.g. information and communication technologies (ICTs) and other areas within electronic industries, where the essential patents related to a standard might be distributed among dozens of actors.
The purpose in this paper is therefore to develop and present a generalized method to calculate reasonable royalties, which works not only in one-to-one but also in many-to-many (as well as in one-to-many and many-to-one) licensing deals.
2. The Investment-Based Method
Companies typically pay dividends to their shareholders in relation to the shareholders' invested capital, i.e. each stock has the same rate of return in form of a dividend. This is a fairness principle that is well established in contemporary business. We argue that this principle for fair and reasonable value-sharing is applicable also in quasi-integrated organizational forms and open innovation (e.g. in licensing collaborations). Hence, fair and reasonable royalties can be calculated by equalizing the rates of returns on the investments made by the parties involved. This principle is then one out of several possible principles for fairness in value-sharing, and based on this principle an investment-based method for royalty determination can be developed.
According to the principle the returns from a licensing deal should be shared among the involved actors in proportion to their respective investment, I. Thus, we employ a FRAND rule that the rate of returns on investments, R, should be equal for all involved actors in order to develop a method for FRAND royalty determination. We start on Case A with only one licensee (buyer), b, and one licensor (seller), s, based on the analysis in Granstrand (2006).2 We then continue with Case B by adding multiple licensees, and subsequently with Case C by adding multiple licensors. Note that we use a separation between total profits, π, operating profits from product/ service sales (not including investment depreciation or license payments), πop, license payments, L, and finally the related investments by the licensor, Is, and licensee, Ib, in R&D, production, and marketing (investments necessary to generate the operating profit). Also note that we, for simplicity reasons, use a one-period approach, which can be easily generalized in principle to a multi-period risk-adjusted approach, however (see Granstrand, 2006).3 Throughout this chapter we use the assumptions that the total operating profits are large enough to cover the total investments (i.e. that the total return on investment for all actors as a collective is positive) and that each licensee's operating profit is large enough to cover its investment. The method is applicable not only to patent licenses, but to intellectual property (IP) licenses in general, including know-how licenses.
The choice of a useful language for presenting a model and its arguments is neither a small issue, nor a small task. Essentially, there are three options in presenting a mathematical model as in our case—using normal text, using symbols, or using a mixture of text and symbols. History provides many examples of how not only disciplines but whole civilizations have been helped or hindered by their choice of mathematical language in their daily practices, be they about economic or engineering or even legal calculations.4 Unfortunately there is a long-lasting divide between economics and law in this respect. We certainly appreciate the need to bridge this divide, and have attempted to do so in this paper by complementing a more precise symbolic presentation of our model with a more accessible but less precise textual presentation.5
2.1 Case A: One Licensor and One Licensee
Case A includes one licensor s with investment Is, and one licensee b with investment Ib and operating profit πopb, and a license royalty payment L. The analysis starts by setting up the expressions for the rates of return on investment for the licensor, Rs, and licensee, Rb, respectively. The rate of return for each party is then the relation between its total profit and its investment. The licensor's total profit, πs, is its received royalties, L, minus its investments, Is. The licensee's total profit, πb, is the operating profit, πopb, minus the royalties, L, paid to the licensor and the investments, Ib:
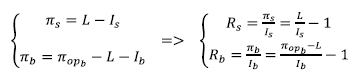
By employing the proposed principle of fairness Rs = Rb (i.e. that the licensor and licensee should receive the same rate of return on investment) we can conclude that the reasonable royalty rate (with operating profit as royalty base) is equal to the licensor's share of the total investments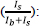 
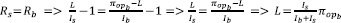
Thus, a share of the licensee's operating profits based on the licensor's share of the total investments should be paid to the licensor as licensing royalty in order to reach a fair and reasonable bilateral licensing agreement:
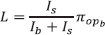
Hence, if the licensor has made e.g. 35% of total investments, 35% of the operating profits should be paid by the licensee to the licensor. A virtue of the method, besides providing a basis for fair valuesharing is then that the objectives of the buyer and seller to maximize profits are aligned, since operating profit is used as royalty base (this is not the case if gross revenue is used as a royalty base).6
2.2 Case B: One Licensor and Multiple Licensees
Case B includes a single licensor s with investment Is, and multiple (m) licensees bi, i = 1, ..., m, with investments Ibi, operating profits πopbi, and license royalty payments Li. 7
By calculations presented in the appendix we can conclude that licensee i should make the following license payment to the licensor:
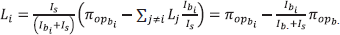
Thus, licensee i should make a payment equal to the size of its operating profit minus a share of the collective operating profits for all licensees. This share of collective operating profits should be equal to the licensee's share of the total investments (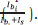
). Notice that this solution in the special case with only one buyer then gives the same solution as in Case A.
2.3 Case C: Multiple Licensors and Multiple Licensees
Case C includes multiple (n) licensors sk, k = 1, ..., n, with investments Isk, and multiple (m) licensees bi, = 1, ..., m, with investments Ibi, operating profits πopbi, and license royalty payments Li k from licensee i to licensor k. This can again (see appendix for Case B) be solved by initially treating the set of licensors and set of licensees, respectively, in a collective way. Thus, the royalties paid by licensee i will again be (now with multiple investing licensors to be paid):
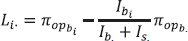
These royalties can be collected in a pool and thereafter distributed to the various licensors (contributors) in accordance with their respective shares of the total licensor investments Is.. Alternatively, the royalties can be collected by the licensors directly from the different licensees, so that a specific licensor k will collect the specific royalty Li k from licensee i:
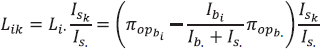
This is thus a general investment-based method for calculating reasonable royalties to be paid from licensee i to licensor k in cases of e.g. standard technologies with multiple essential patents. Notice that this solution in the special case with only one licensor then gives the same solution as in Case B, and in the special case with only one licensor and one licensee the same solution as in Case A.
2.4 Summary of The Investment-Based Method
The algebra resulting in the generalized investmentbased method above may seem complex, but it results in a simple and easy-to-use method, based only on the involved actors' operating profits and investments. The table below can be used in practice to calculate the royalties to be paid and collected by various actors in a multilateral royalty agreement. First, determine for each licensor and licensee their relevant amounts invested. Second, determine for each licensee their relevant operating profits related to the licensed IP. Third, determine the FRAND royalties to be paid by each licensee, and the amount that should be received by each licensor, either by using the formula above or the table below. The fairness principle to equalize all parties' rates of returns on investment then leads to license fees or royalties expressed as amounts in monetary units rather than royalty rates expressed as percentages of some royalty base (see the example in the next section).
Notice that a licensor might very well be also a licensee (which is most often the case in licensing standard technologies), which introduces no additional problems to the presented method. If using the table below for calculations, such an actor, being both licensor and licensee, should be included both as a seller and buyer, and the investments need to be divided into seller- and buyer-related (although this relative division does not impact the total return for such an actor—and does thereby not introduce any additional problems). A more extensive spreadsheet for the calculations to be used in practice can be found at www.ip-research.org.
2.5 An Illustrative Example
Assume that two different firms, s1 and s2, have developed different technologies that jointly (but not separately) enable a new product area within automotive security. They have invested $5M and $4M in R&D, respectively. None of these firms have production and marketing capabilities, however, and they therefore decide to license out their technologies to three different incumbent firms, b1, b2, and b3, active in producing and selling automotive security products. Due to their various production and marketing capabilities, these firms need to make investments of $1M, $3M, and $2M, respectively, to enable operating profits of $7M, $10M, and $11M, respectively. Considering these numbers and using the table on pg. 191 we can now use the investmentbased method to calculate the royalty payments, see table below. Notice the comparison with the 25% rule of thumb (based on operating profits minus investments as royalty base, considering that the definition of operating profit does not include investment depreciation in this paper), which results in a large variety of rates of return on investment.
| Royalties Worksheet | A | B | C | D | E |
| | Seller/Licensor | | Investment | Royalty Income | Profit | Rate of RoI |
| 1 | | | Fill in | C10×(B1/B5) | C1-B1 | D1/B1 |
| 2 | | | Fill in | C10×(B2/B5) | C2-B2 | D2/B2 |
| 3 | | | Fill in | C10×(B3/B5) | C3-B3 | D3/B3 |
| 4 | | | Fill in | C10×(B4/B5) | C4-B4 | D4/B4 |
| 5 | Total | | Sum | Sum | Sum | D5/B5 |
| | | A | B | C | D | E |
| | Buyer/Licensee | Operating Profit | Investment | Royalty Payment | Profit | Rate of RoI |
| 6 | | Fill in | Fill in | A6-A10×B6/(B5+B10) | A6-B6-C6 | D6/B6 |
| 7 | | Fill in | Fill in | A7-A10×B7/(B5+B10) | A7-B7-C7 | D7/B7 |
| 8 | | Fill in | Fill in | A8-A10×B8/(B5+B10) | A8-B8-C8 | D8/B8 |
| 9 | | Fill in | Fill in | A9-A10×B9/(B5+B10) | A9-B9-C9 | D9/B9 |
| 10 | Total | Sum | Sum | Sum | Sum | D10/B10 |
3. Discussion, Generalizations and Limitations
Previous literature about the 25% rule has discussed a) to what extent the rule is based on heuristics (e.g. as a rule of thumb), conventional wisdom, and/or empirical statistics, b) whether the rule is generally applicable or limited only to special cases, and c) what would be the basis for the rule, EBITDA or something else (see e.g. Goldscheider, 2011, and Kidder and O'Brien, 2011). This literature discussion has also pointed at a number of pros and cons of the rule, which by and large has led to interpreting the rule more as a flexible guideline or methodology (the classic 25% rule) than a rigid rule for determining royalty rate and royalty base across industries and business situations (the 25% rule of thumb).
However, previous literature has, despite its normative underpinnings, rarely presented any evidence-based alternative to the 25% rule, let alone an alternative with more general applicability. This paper has presented an alternative; a generalized investment-based method to determine fair and reasonable royalties in the case of one or many buyers of non-exclusive licenses from one seller or from a consortium of many sellers pooling their patents and know-how.
The question then is: What are the generalizations and limitations of this method of determining FRAND terms? A few points can be made here.
First, in case an improvement of an underlying technology is made based on an investment ΔI= I1 – I0, separable from the original investment I0, and this improvement has an added value ΔV= V1 – V0, separable from the original value V0, then a second round of FRAND terms could be determined with respect to ΔV, ΔI, and related previous investments sI0. In a similar way the rates of return on investment for the relevant parties are equalized with respect to their shares of added value ΔV and their respective shares of the relevant investments (ΔI + sI0, where s is the share of previous investments leading to the added value in a complementary way with ΔI). In case of a patent pool newcomers will thus not be favored or free-riding. On the contrary, there is an incentive to join the pool at an early stage, and early mover advantages could thus be designed by the original pool members by defining the relevant investment base for latecomers, e.g. in terms of essential patents.
Second, if the FRAND licensing arrangement concerns a component, feature, or subsystem of a larger product or system, similar principles as for an improvement could be applied, assuming that relevant investments (or costs) and returns (or revenues) could be identified.
Third, if e.g. the licensor wants to impact the industrial organization of the sellers, a somewhat modified method can be used in which the rates of returns on investments for licensors and licensees as collectives are equalized, while the individual rates of returns on investments for various licensees are allowed to vary. Such a setup then promotes competition among the licensees (product/service sellers), for instance in order to mitigate anti-trust risks or to promote efficiency. Other modifications can be made in order to promote collaboration, for instance in order to promote collective learning (as was the case in the licensee family of VHS).
As to limitations of the investment-based method, a few could be pointed out here. Differences in R&D productivity are not taken into account, e.g. essential vs. non-essential patents. There are many ways to insert correction factors for such differences, however. Notice especially that the given method might create incentives for unnecessary high investments ex ante (since profits are later shared in accordance with investment levels), which is why adjustments might need to be made for the productivity of each actor's investments. Further, synergies and substitutes across businesses and technologies are not taken into account (note e.g. that two essential patents could be substitutes, so that they are jointly non-essential). Again, there are methods to deal with such situations (e.g. by focusing on incremental operating profits for the collective as a whole), albeit cumbersome, and the method proposed here then serves as a first approximation.
The method in an extended form allows for a dynamic perspective, as described above. This introduces boundary conditions, however, since new entrants should not imply lower profits for any of the already included members (which might be the case if a newcomer provides a positive incremental profit, but with a lower rate or return on investment than the one obtained by the original collective). Additional boundary conditions might need to be considered in cases of infringement, e.g. that the incremental total profit (considering also cannibalism on infringed sales) should be shared at the same time as the licensor (plaintiff) should be at least as profitable as without infringement, but this depends on the jurisdiction.
| The Investment-Based Method | Comparison With The 25% Rule Of Thumb |
| Seller/ Licensor | | Investment | Royalty Income | Profit | Rate of RoI | Royalty Income | Profit | Rate of RoI |
| s1 | | 5 | 9.33 | 4.33 | 87% | 5.5 | 0.5 | 10.0% |
| s2 | | 4 | 7.47 | 3.47 | 87% | 5.5 | 1.5 | 37.5% |
| Total | | 9 | 16.8 | 7.80 | 87% | 11 | 2 | 22.2% |
| The Investment-Based Method | Comparison With The 25% Rule Of Thumb |
| Buyer/ Licensee | Operating Profit | Investment | Royalty Payment | Profit | Rate of RoI | Royalty Payment | Profit | Rate of RoI |
| b1 | 7 | 1 | 5.13 | 0.87 | 87% | 3 | 3 | 300% |
| b2 | 10 | 3 | 4.40 | 2.60 | 87% | 3.5 | 3.5 | 117% |
| b3 | 11 | 2 | 7.27 | 1.73 | 87% | 4.5 | 4.5 | 225% |
| Total | 28 | 6 | 16.8 | 5.20 | 87% | 11 | 11 | 183% |
4. Summary and Conclusions
The received 25% rule of thumb is only applicable in a very special case, and then only in case of bilateral licensing, and should consequently be dismissed as a general rule, just as the CAFC did. The method is simple, but overly so, and its widespread use in the past does not justify its use in the future. More accurate methods are called for, not the least in light of the evolving pro-patent and pro-licensing era (Granstrand, 1999, 2004, 2012) with larger and more rapid deal flows with more complex deal structures, calling for more sophisticated management tools. The investment-based method proposed in this paper offers a new approach to royalty determination in FRAND terms that results in equal rates of return on investments. This method is not only applicable to one-to-one licensing deals, but is also applicable to non-exclusive multi-lateral licensing as well as to patent pools and other forms of open innovation, using licensing for governance. The method is far from the final say in these situations, but rather a first step in a promising direction, hopefully leading to decreased transaction costs from e.g. bargaining and litigation. More developments are needed by analysts and practitioners, however. Court decisions raising the bar and burden of proof are welcome in this process. Old methods, just as old technologies, sooner or later have to say farewell and be gracefully acknowledged.
Appendix: Calculations
Case B includes one licensor s with investment Is, and many (m) licensees bi, i = 1, ..., m, with investments Ibi, operating profits πopbi, and license royalty payments Li. We start by repeating the analysis in Case A:
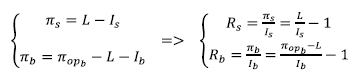
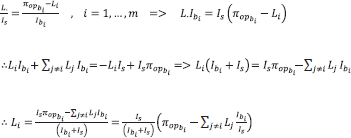
Although this introduces additional unknown variables to the equation (Lj), this can be solved by considering that all licensees can be treated collectively, as well as individually, meaning that the rate of return on investment should be the same for the licensor, the licensee, and all licensees as a collective:
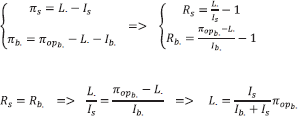
Now, using the relation L. = Li + Σj≠iLj it is possible to create an expression for Li without any unknown variables:
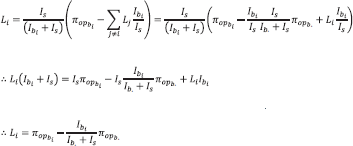
Acknowledgements
The work with this paper has been conducted under the auspices of IMIT (Institute for Management of Innovation and Technology) at Chalmers University of Technology within the project “Management, Economics and IP Law of Open Distributed Innovation Processes.” The financial support from the European IP consultancy firm Zacco, the German energy corporation E.ON, and the Swedish government research foundation Vinnova is gratefully appreciated.
References
Al-Khalili, J. (2010) Pathfinders: The Golden Age of Arabic Science, Penguin Books Ltd.
Epstein, R.J. and Marcus, A.J. (2003) “Economic Analysis of the Reasonable Royalty: Simplification and Extension of the Georgia-Pacific Factors,” Journal of the Patent & Trademark Office Society, Vol. 85, No. 7, pp. 555-583.
Goldscheider, R. (2011) “The Classic 25% Rule And The Art Of Intellectual Property Licensing.” les Nouvelles, September 2011.
Goldscheider, R. (2012) “The Current Realities Of The Classic 25% Rule: An Attempt To Put The House In Order,” les Nouvelles, March 2012.
Granstrand, O. (1999) The Economics and Management of Intellectual Property: Towards Intellectual Capitalism, Cheltenham: Edward Elgar Publishing.
Granstrand, O. (2004) “The economics and management of technology trade: towards a pro-licensing era?,” International Journal of Technology Management, Vol. 27, Nos. 2/3, pp. 209-240.
Granstrand, O. (2006) “Fair And Reasonable Royalty Rate Determination—When Is The 25% Rule Applicable?,” les Nouvelles, September 2006.
Granstrand, O. (2012) Patents and Innovation for Growth and Welfare, Cheltenham: Edward Elgar Publishing.
Granstrand, O. and Holgersson, M. (forthcoming) “Multinational technology and intellectual property management—Is there global convergence and/or specialization?” forthcoming in International Journal of Technology Management.
Granstrand, O., Patel, P. and Pavitt, K. (1997) “Multi-Technology Corporations: Why They Have “Distributed” Rather Than “Distinctive Core” Competences,” California Management Review, Vol. 39, No. 4, pp. 8-25.
Holgersson, M. (2011) Intellectual Property Strategies and Innovation: Causes and Consequences for Firms and Nations, Licentiate Thesis, Chalmers University of Technology.
Kemmerer, J.E. and Lu, J. (2008) “Profitability and Royalty Rates Across Industries: Some Preliminary Evidence,” Journal of the Academy of Business and Economics, March 2008.
Kidder, D.G. and O'Brien, V.E. (2011) “Simply Wrong: The 25% Rule Examined,” les Nouvelles, December 2011.
Lu, J. (2011) “The 25% Rule Still Rules: New Evidence From Pro Forma Analysis in Royalty Rates,” les Nouvelles, March 2011.
Pratt, J.W. and Zeckhauser, R.J.(1985) ‘Principals and agents: An overview,” In Pratt, J.W. and Zeckhauser, R.J. (Eds.) Principals and agents: The structure of business, Cambridge: Harvard Business School Press.
Razgaitis, R. (1999) Early-Stage Technologies: Valuation and Pricing, New York: John Wiley & Sons, Inc.
Ruikka, T. (2008) ‘“Frand” Undertakings In Standardization— A Business Perspective,” les Nouvelles, September 2008.
Sherry, E.F. and Teece, D.J. (2004) “Royalties, evolving patent rights, and the value of innovation,” Research Policy, Vol. 33, No. 2, pp. 179-191.
Smith, G.V. and Parr, R.L. (1998) Intellectual Property: Licensing and Joint Venture Profit Strategies, New York: John Wiley & Sons, Inc.
Struik, D.J. (1987) A concise history of mathematics (4th ed.), New York: Dover Publications, Inc.
Tversky, A. and Kahneman, D. (1974) “Judgment under Uncertainty: Heuristics and Biases,” Science, Vol. 185, No. 4157, pp. 1124-1131.
- Uniloc U.S.A., Inc. v. Microsoft Corp., 632 F.3d 1292 (Fed. Cir. 2011).
- Granstrand (2006) also showed that the 25% rule of thumb is only applicable in a very special case.
- Uncertainty, risk, and time preferences (including financial discounting) can be dealt with as in Granstrand (2006).
- See e.g. Struik (1987) or Al-Khalili (2010).
- On a concluding note, one may observe in all fairness how textual representations easily become cumbersome, just as algebraic calculations became cumbersome for ancient Greeks and Romans with their notation of mathematical relations. Making the textual explanations less cumbersome usually implies a loss of precision with ensuing misrepresentations. Hopefully a mix of text and symbols are reasonable and non-discriminatory to the readers.
- The so called principal-agent problem arguably includes two important aspects; information asymmetries and unaligned incentives (see e.g. Pratt and Zeckhauser, 1985). In the case of licensing deals and the choice of royalty bases, the use of operating profits aligns the incentives of the licensee and the licensor on one hand, but the licensee's operating profits may on the other hand be less easily monitored than its revenues by the licensor, leading to information asymmetries.
- Notice that the following convention is used throughout: x. = Σi xi